Quadratic Equation Definition Formulas and History

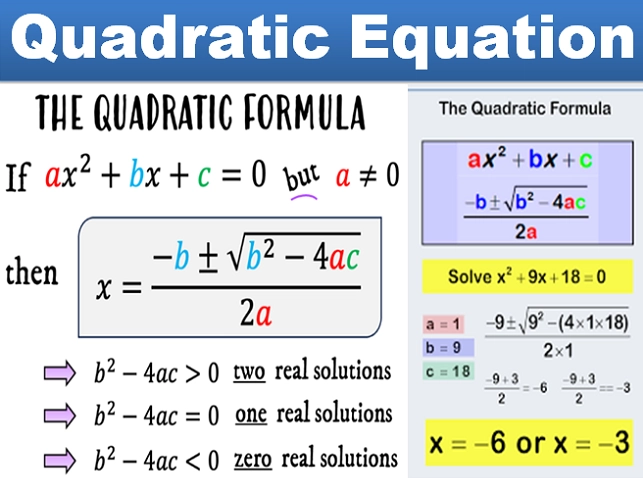
The Quadratic formula is a fundamental mathematical tool used to solve quadratic equations. Quadratic equations are equations of the form ax² + bx + c = 0, where “a,” “b,” and “c” are coefficients, and “x” is the variable we aim to solve for. This formula provides a systematic way to find the solutions (roots) of such equations, which can be real or complex numbers. In this comprehensive guide, we will delve into the details of the quadratic formula, explore its origins, and provide examples of its applications.

Historical Context

Before we explain the Quadratic formula in detail, let us look at some historical context to understand how humans through the ages have approached the problem of solving quadratic equations.

Ancient Methods

The origins of quadratic equations date back thousands of years to ancient civilizations like the Babylonians and Egyptians. They had methods for finding approximate solutions to quadratic equations but lacked the formal algebraic notation we use today.

The Greek Influence

In ancient Greece, mathematicians like Euclid, Diophantus, and Archimedes worked on various aspects of algebraic equations, including quadratic equations. However, they often solved these equations geometrically rather than algebraically.

Renaissance and European Developments

In the Renaissance, European mathematicians like Viète and Descartes contributed to the development of algebra, laying the groundwork for the formalization of algebraic techniques. However, it wasn’t until the 16th century that the quadratic formula, as we know it today, was derived by European mathematicians.

Read Also

Mathematics Definition History & Importance Branches

The Quadratic Formula

The quadratic formula is a mathematical expression that provides a convenient and systematic way to find the solutions of a quadratic equation. It’s derived from completing the square, a technique that allows us to rewrite a quadratic equation in the form (x – h)² = k. This form makes it easy to isolate “x” and find the solutions.

The quadratic formula is as follows:

$$x = \frac{-b \pm \sqrt{b^2 – 4ac}}{2a}$$

In this formula:

– “a,” “b,” and “c” are coefficients of the quadratic equation ax^2 + bx + c = 0. – The symbol “” indicates that there are typically two solutions: one with a plus sign and another with a minus sign. – The term inside the square root, b^2 – 4ac, is known as the discriminant.

III. Using the Quadratic Formula

To solve a quadratic equation using the quadratic formula, follow these steps:

1. Identify the coefficients “a,” “b,” and “c” from the given quadratic equation.

2. Substitute these values into the quadratic formula:

$$x = \frac{-b \pm \sqrt{b^2 – 4ac}}{2a}$$

3. Compute the discriminant (the value inside the square root): $D = b^2 – 4ac$.

4. If the discriminant is positive (D > 0), there are two real solutions. If it’s zero (D = 0), there’s one real solution (a repeated root). If it’s negative (D < 0), there are two complex solutions.

5. Calculate the two solutions using the formula. For a positive discriminant:

$$x_1 = \frac{-b + \sqrt{D}}{2a}$$ $$x_2 = \frac{-b – \sqrt{D}}{2a}$$

For a discriminant of zero:

$$x_1 = x_2 = \frac{-b}{2a}$$

For a negative discriminant:

$$x_1 = \frac{-b}{2a} + \frac{\sqrt{-D}}{2a}i$$ $$x_2 = \frac{-b}{2a} – \frac{\sqrt{-D}}{2a}i$$

6. These values represent the solutions to the quadratic equation.

IV. Examples

Let’s work through a few examples to illustrate how the quadratic formula is used to find the solutions of quadratic equations.

Example 1: Simple Quadratic Equation Solve the equation $2x^2 – 5x + 2 = 0$ using the quadratic formula.

In this equation, we have: – $a = 2$ – $b = -5$ – $c = 2$

Now, apply the quadratic formula:

$$x = \frac{-(-5) \pm \sqrt{(-5)^2 – 4 \cdot 2 \cdot 2}}{2 \cdot 2}$$

Simplify:

$$x = \frac{5 \pm \sqrt{25 – 16}}{4}$$

Now, calculate the solutions:

$$x_1 = \frac{5 + \sqrt{9}}{4} = \frac{5 + 3}{4} = \frac{8}{4} = 2$$

$$x_2 = \frac{5 – \sqrt{9}}{4} = \frac{5 – 3}{4} = \frac{2}{4} = 0.5$$

So, the solutions to the equation $2x^2 – 5x + 2 = 0$ are $x = 2$ and $x = 0.5$.

Example 2: Repeated Root Solve the equation $x^2 – 4x + 4 = 0$ using the quadratic formula.

In this equation, we have: – $a = 1$ – $b = -4$ – $c = 4$

Apply the quadratic formula:

$$x = \frac{-(-4) \pm \sqrt{(-4)^2 – 4 \cdot 1 \cdot 4}}{2 \cdot 1}$$

Simplify:

$$x = \frac{4 \pm \sqrt{16 – 16}}{2}$$

Here, the discriminant is zero ($D = 0$), which means we have a repeated root.

$$x_1 = \frac{4 + \sqrt{0}}{2} = \frac{4}{2} = 2$$

$$x_2 = \frac{4 – \sqrt{0}}{2} = \frac{4}{2} = 2$$

The equation $x^2 – 4x + 4 = 0$ has one real solution: $x = 2$.

Example 3: Complex Solutions Solve the equation $x^2 + 2x + 5 = 0$ using the quadratic formula.

In this equation, we have: – $a = 1$ – $b = 2$ – $c = 5$

Apply the quadratic formula:

$$x = \frac{-2 \pm \sqrt{2^2 – 4 \cdot 1 \cdot 5}}{2 \cdot 1}$$

Simplify:

$$x = \frac{-2 \pm \sqrt{4 – 20}}{2}$$

Here,

the discriminant is negative ($D = -16$), which means we have complex solutions.

$$x_1 = \frac{-2}{2} + \frac{\sqrt{-16}}{2}i = -1 + 2i$$ $$x_2 = \frac{-2}{2} – \frac{\sqrt{-16}}{2}i = -1 – 2i$$

The equation $x^2 + 2x + 5 = 0$ has two complex solutions: $x = -1 + 2i$ and $x = -1 – 2i$.

Quadratic Equation

A quadratic equation is a second-degree polynomial equation, which means the highest power of the variable (usually denoted as “x”) in the equation is squared. It has the general form:

ax² + bx + c = 0

Where:

• “a,” “b,” and “c” are coefficients, and “a” must be a nonzero value. These coefficients are typically real numbers.

The main goal when working with a quadratic equation is to find the values of “x” that satisfy the equation, making it equal to zero. These values of “x” are called the solutions or roots of the quadratic equation.

Quadratic equations can have one of three types of solutions, depending on the discriminant (the value inside the square root in the quadratic formula):

1. Two Real and Distinct Solutions: If the discriminant (b² – 4ac) is positive, the quadratic equation has two different real solutions. These solutions are real numbers, and the graph of the equation is a parabola that intersects the x-axis at two distinct points.
2. One Real Solution: If the discriminant is equal to zero (b² – 4ac = 0), the quadratic equation has one real solution. This solution is a repeated root, and the graph of the equation touches the x-axis at a single point.
3. No Real Solution: If the discriminant is negative (b² – 4ac < 0), the quadratic equation has no real solutions. In this case, the solutions are complex numbers, and the graph of the equation does not intersect the x-axis.

To find the solutions of a quadratic equation, you can use the quadratic formula:

$$x = \frac{-b \pm \sqrt{b^2 – 4ac}}{2a}$$

Here’s a step-by-step guide to solving a quadratic equation using the quadratic formula:

1. Identify the coefficients “a,” “b,” and “c” from the given quadratic equation.
2. Calculate the discriminant (Δ) using the formula: Δ = b² – 4ac.
3. Determine the nature of the solutions based on the value of the discriminant:

• If Δ > 0, there are two real and distinct solutions.
• If Δ = 0, there is one real solution (a repeated root).
• If Δ < 0, there are no real solutions.

1. Use the quadratic formula to calculate the solutions by substituting the values of “a,” “b,” and “c” into the formula. You will typically have two solutions: x₁ and x₂.
2. If necessary, simplify the solutions, especially if they involve square roots or complex numbers.

Quadratic equations are fundamental in algebra and have numerous applications in various fields, including physics, engineering, and economics. They describe the behavior of many natural phenomena and are a key concept in mathematics.

Conclusion

The quadratic formula is a powerful mathematical tool that provides a systematic way to find the solutions of quadratic equations. Whether the solutions are real, repeated, or complex depends on the discriminant of the equation. Throughout history, mathematicians from various cultures have contributed to the development of techniques for solving quadratic equations, culminating in the elegant quadratic formula we use today. It continues to be a fundamental concept in mathematics and finds applications in various fields, including physics, engineering, and computer science. Understanding how to use the quadratic formula is essential for solving a wide range of problems in these disciplines and beyond.